Compound interest has been called the eighth wonder of the world. We cannot verify that, but it is a reliable way to watch your money grow while you do very little.
This guide explains what compound interest is, how it works, and how to calculate it. It also covers how compound interest differs from simple interest, and how tools like the rule of 72 help you estimate your growth — with Canadian examples throughout.
What is compound interest?
Compound interest is the interest you earn on both your original money and on the interest it has already earned. Because your interest earns interest, your balance grows faster the longer you leave it. The larger your balance gets, the bigger each interest payment becomes.
Compound interest definition
Compound interest is a way of calculating interest where the interest earned over time is added back to the principal sum. You earn interest on the principal, and then you earn interest on that interest. This happens because you save your interest rather than withdrawing it.
Compound interest generally applies to savings accounts and loans. You agree to borrow or save money over a set period, and interest accumulates and compounds during that time. It is applied on a set frequency — daily, weekly, quarterly, or annually.
How does compound interest work?
Compounding is popular in saving and investing because it helps you calculate the future value of your money. Watching your balance grow exponentially is a good feeling, too. The interest-on-interest effect can generate meaningful sums when you are patient and keep contributing.
Because it projects the future value of your savings, compounding helps you plan. You can work out how long you need to save, and how much to contribute, to reach a specific goal. That might be a deposit on your first home, or a wedding.
Here is an example of how compounding can grow your money. Say you invest $10,000 for 3 years at a return of 5.5% compounded annually. Each year the earnings grow, and by the end of the third year your balance is worth $11,742.41.
Year | Beginning of year value | Yearly Earnings | End of year value |
|---|---|---|---|
| 1 | $10,000 | $550 | $10,550 |
| 2 | $10,550 | $580.25 | $11,130.25 |
| 3 | $11,130.25 | $612.16 | $11,742.41 |
Compound interest is not limited to savings and investment accounts. Chequing accounts and guaranteed investment certificates (GICs) work on the principle of compound interest, too. Assets like stocks, mutual funds, and exchange-traded funds (ETFs) can accrue returns, which is why investment accounts experience compounding.
Your money can be compounded daily, weekly, monthly, or yearly. The more often it is compounded, the faster it grows — this is called the compounding frequency.
A common way to compare interest rates is the annual equivalent rate (AER). This figure shows what the annual rate would be if interest were paid for a full year and compounded. It is sometimes called the annual percentage yield (APY).
How to calculate compound interest
Compound interest formula
Interest earned = P (1 + i/n)^nt – P
Here is what each variable means:
P — the principal amount, or present value: the starting amount of your savings or the total value of a loan.
i — the interest rate expressed as a decimal (5% = 0.05).
n — the number of times the interest is compounded each year.
t — the number of years the interest will be applied.
Confused? Fair enough. Here is how to work through it for a savings account with $15,000 that earns 5% annual interest for 30 years.
Interest earned = $15,000 (1 + .05/1)^1(30) – $15,000
Interest earned = $15,000 (1.05)^30 – $15,000
Interest earned = $15,000 (4.32) – $15,000
Interest earned = $64,800 – $15,000
Interest earned = $49,800
Not so bad.
Compound interest vs. simple interest
We have focused on compound interest, but it helps to understand its foundation: simple interest. Simple interest is the interest an initial sum earns over time, without adding interest on the interest already earned.
Simple interest is found by multiplying the daily interest rate by the original amount, then by the number of days between payments. It is common for basic loans, and is usually measured with the annual percentage rate (APR), which shows the interest for a whole year.
Here is how simple and compound interest compare. In this example, you have $100 in the bank at an interest rate of 5% per year.
First, simple interest:
Year 1: $100 + $5 = $105 ($5 in interest)
Year 2: $105 + $5 = $110 ($5 in interest)
Year 3: $110 + $5 = $115 ($5 in interest)
Now compound interest:
Year 1: $100 + 5% = $105 ($5 in interest)
Year 2: $105 + 5% = $110.25 ($5.25 in interest)
Year 3: $110.25 + 5% = $115.76 ($5.51 in interest)
The rule of 72
Say you are happy with your rate of return and want to know how long it would take to double your money. You could do a long calculation, or use the handy rule of 72.
The rule says that 72 divided by the annual interest rate gives the number of years to double your money, without contributing another cent. It is useful for quick mental math, and for picturing the impact of compound interest.
Say you have about $10,000 invested and want to know how long it will take to double. At an interest rate of 5%, it would take roughly 14 years.
Compounding frequency
As noted, the compounding frequency is the number of times per year that accumulated interest is credited to the account. Some institutions credit it monthly or quarterly instead. The greater the compounding frequency, the more opportunity your money has to grow.
For example, say you invest $5,000 at a 5% interest rate. Compounded monthly, you would have $5,255 at the end of the first year; compounded annually, you would have $5,250. The gap looks small over 1 year, but it adds up over time.
Continuous compound interest
We have covered simple interest and compound interest. Continuous compounding is the natural conclusion: what would happen if interest kept compounding without a deadline. Think of it as compounding taken to its theoretical limit.
Instead of calculating for a set number of years or months, you assume constant compounding over an infinite number of periods. It is not possible in practice, but it is an important theoretical concept in finance. Here is the formula:
= P x e (i x t)
Here is what each variable means:
e — the mathematical constant, approximately 2.7183.
i — the stated interest rate.
t — the time period, in years.
Benefits of compound interest
The main benefit of compound interest is that you earn more thanks to the compounding effect. If you reinvested your returns on $10,000 over 30 years and earned 5.5% interest, you would end up with nearly 5 times that amount. Each year your balance increases, and so do your returns.
The money you have made makes money. This snowball effect is a powerful way to build wealth over time. It is one reason to resist withdrawing from your savings and investment accounts, and to aim for a longer time horizon.
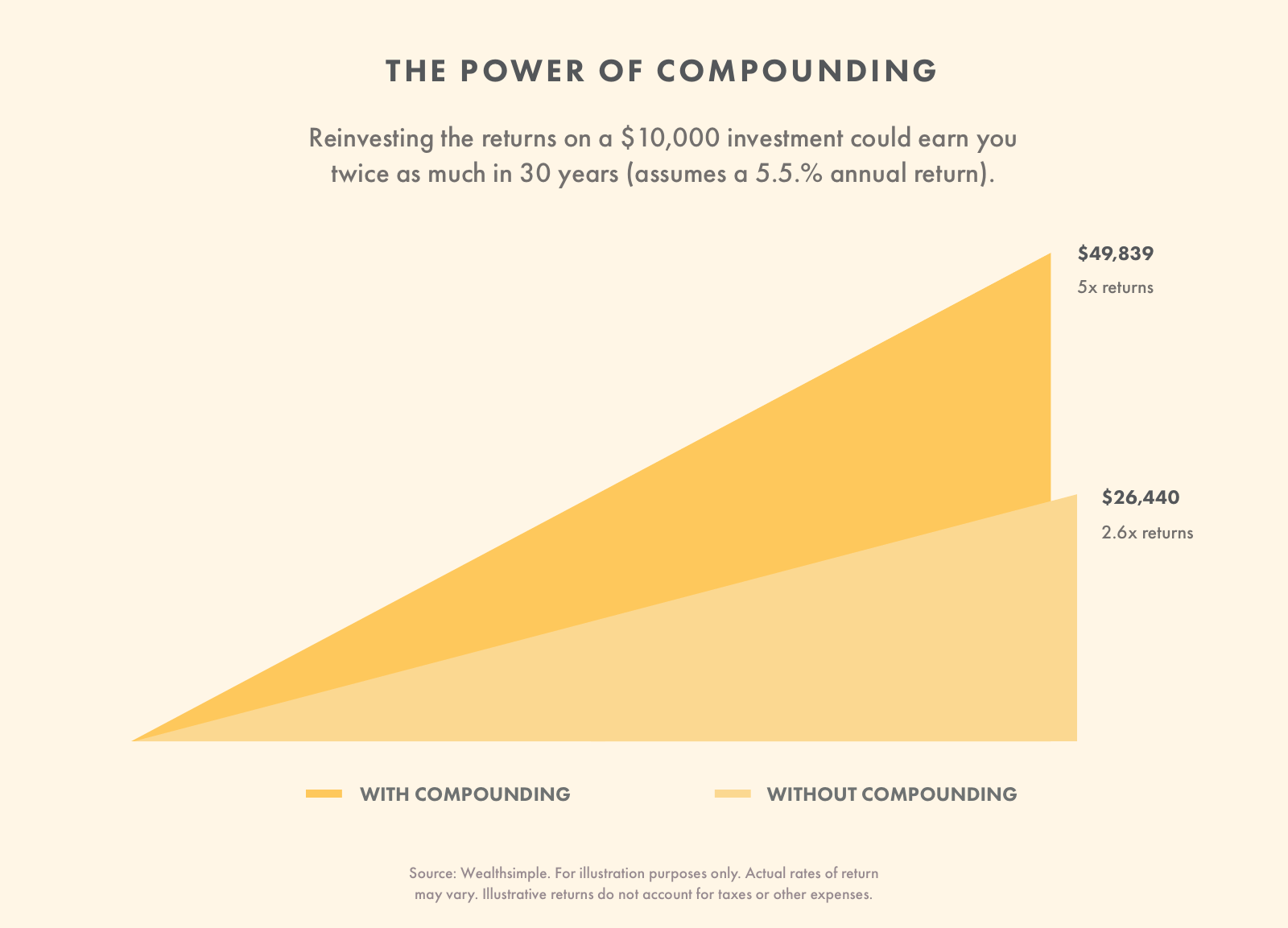
When you put money into an interest-earning account, each period's interest is added to your balance. That new balance is used to calculate the next interest payment. Because your balance keeps growing, so does the interest you earn.
A steady approach helps: make regular contributions and leave the money untouched until retirement.
Every dollar you add creates a little more interest, and every bit of interest builds your balance. This cycle is how many people build significant wealth. Watching interest grow in a savings account or investment product can be captivating — your money increases without any additional labour.
Keeping money under your mattress is technically saving for the future, but it does not compare to a high-interest savings account or investment portfolio. Without compounding, you would have to work for every dollar of your nest egg.
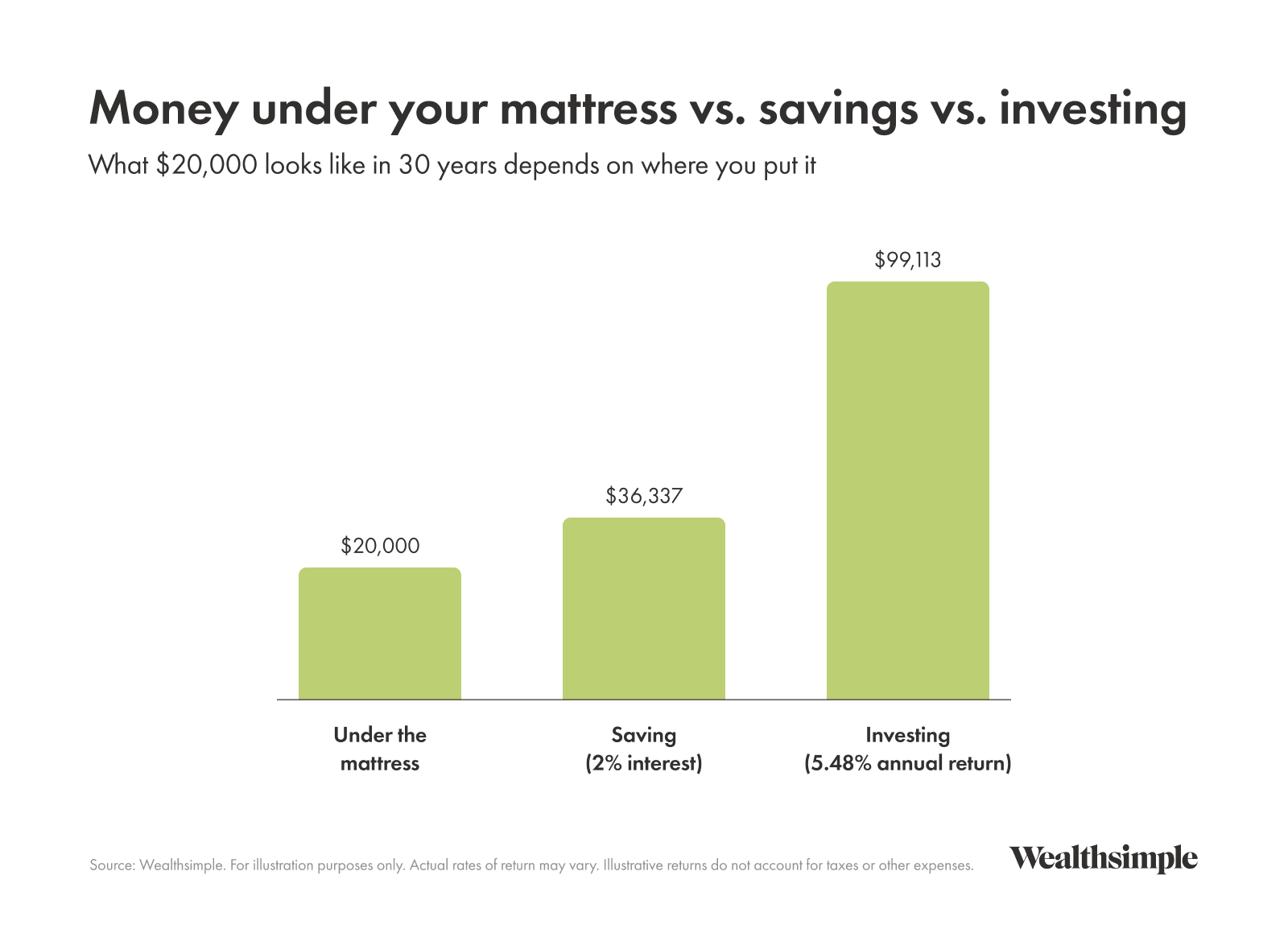
The earlier you start saving, the more you benefit from compounding. As the numbers get bigger, so does the advantage. Think of a snowflake turning into a snowball — the longer the hill, the bigger it grows.
Drawbacks of compound interest
Compound interest works in your favour when you are earning it, but it works against you when you owe money. All that accumulating interest can become a heavy burden if it is debt you are building. When it comes to debt, the interest hurts you, the borrower.
When you take on debt, interest keeps accumulating until the original amount plus interest is paid off. That interest also earns interest, which can turn into a difficult cycle.
Credit cards are a good example of how compound interest can work against borrowers. Assuming you never pay your bill, here is what would happen:
Year 1: $10,000 + 20% interest = $12,000 ($2,000 in interest)
Year 2: $12,000 + 20% interest = $14,400 ($2,400 in interest)
Year 3: $14,400 + 20% interest = $17,280 ($2,880 in interest)
Within 3 years, the amount owed is almost double the original balance. That is why it helps to pay off your credit card balance in full each month. In short: compound interest is helpful when you are earning, and costly when you owe.
Using a compound interest calculator
If you want to skip the formulas, there are free online compound interest calculators. The Ontario Securities Commission offers free financial calculators that show how your money will grow. You enter your initial investment, monthly contributions, the number of years, and the estimated annual interest rate.
Compound interest helps registered accounts like Tax-Free Savings Accounts (TFSAs) and Registered Retirement Savings Plans (RRSPs) grow. Using calculators for these accounts helps you understand how much to contribute for your savings to grow in the future.
